How Rare is Rare? — Playing the NFT lottery with Neolastics.
Neolastics.com is a digital art project that can automatically mint and burn unique, psuedo-randomly generated Mondrian inspired cubes of collectible digital art. What sets this NFT project apart is its bonding curve where you can mint at an incrementing price, and a reward for burning pieces. But one question remains, how rare is rare? And are those elusive green squares the goal? Or is there something even more elusive waiting to be uncovered. A full house equivalent better than 4 of a kind? And how much gas do I need to mine it?
Lets find out, shall we?!
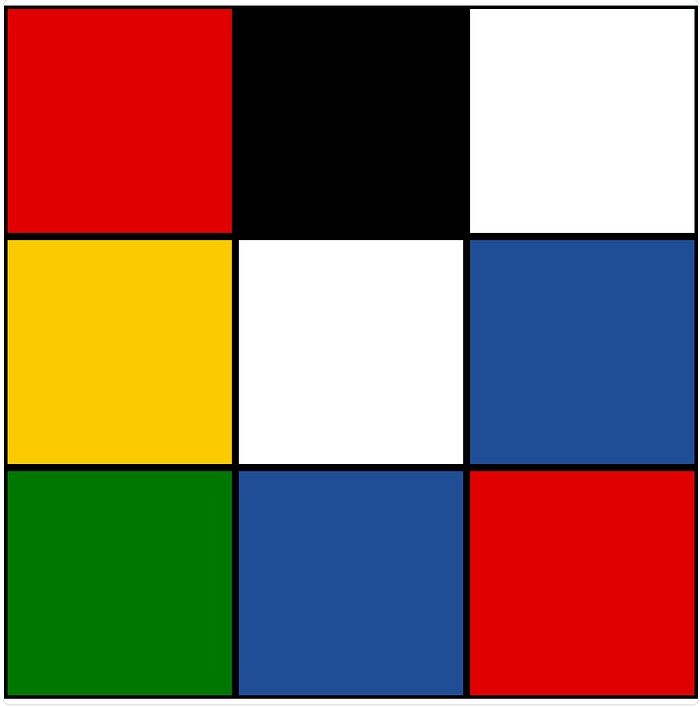
Rules of the Game
- The price curve is linear, starting at 0.001 ETH for a Neolastic piece. Each new piece increases the price by 0.001 ETH.
- 99.5% of the price is kept in the bonding curve reserve. 0.5% goes to the creator.
- There exists 6 colours, with white, black, red, blue, and yellow being equally likely (~20%).
- Green is rare (~1/256).
- The colours are chosen from a psuedo-randomly generated 32 byte hash.
- A maximum of 10,077,696 (6⁹) potential combinations can thus exist.
The elusive Green Square
First thing everyone sees is the ~1/256 and tries their luck. With 9 squares to hit the jackpot, there is ~1/29 chance to add one to your collection. If you have minted less that 29, consider yourself a winner! But did you burn something much more valuable along the way?
1 green = ~1/29
Putting a price on rarity
Now how much is something ultra rare worth?
Fixed gas cost = burn (68,792 wei) + mint (190,912 wei)
Bonding curve cost = 0.5%
At a gas price of 100 wei and token price of 0.5 ETH
1 Green = ~1/29
= 29 x (0.0217 ETH+ 0.5% 0.5ETH ) + 0.5 ETH
= 1.2 ETH ( 2.4x premium)
Or something more difficult, like a full house?
Full house = ~1/1162
= 28.6 ETH ( 57x premium )
Looking for Gems
There are a number of other combinations that much rarer and probably worth keeping besides just a green. Just as you wouldn't throw away four 4’s chasing another Ace in poker, or that a full house is better than a flush, we can do the same for our Neolastics. Well go through some of the probability, save you from the scary math and let you know if it is better than getting a green, and if you should hold onto it.
5+ of a Kind
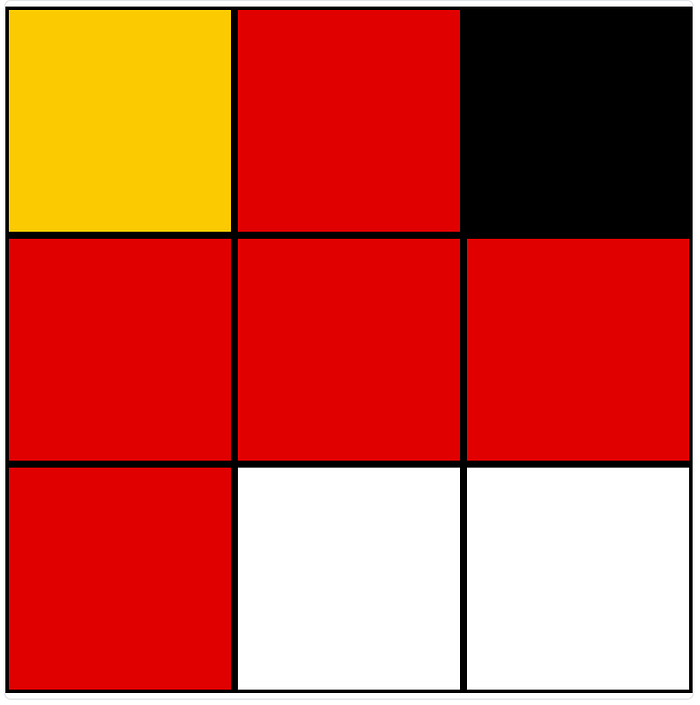
This would be if there were a number of the same color, not including green (we’ll get to that later)
4 of a kind = ~1/3
5 of a kind =~1/12
6 of a kind =~1/72
7 of a kind =~1/678
8 of a kind =~1/10850
9 of a kind =~1/390625
Green Combos
Is this rarer than a full house? It starts to get interesting if you consider combining something rare, with an already rare green square.

Combos
4 of a kind + green = ~1/612
5 of a kind + green = ~1/2448
6 of a kind + green = ~1/14,688
7 of a kind + green = ~1/138,312
Full House
Some of the more aesthetic combinations that have been minted, have only 2 or three colors. The following calculations are if you had two colors, in different ratios.

Full house — 2 Colours
So far, the above full house with 6:3 is the rarest Neolastic minted.
5:4 = ~1/775
6:3 = ~1/1,162
7:2 = ~1/2,712
8:1 =~1/10,850
Gas cost for the Black/Yellow Full house (6:3)
= 1162 x (gas+ 0.5%)
= 28.6 ETH ( 57x premium @ 100 wei, 0.5 ETH/token)
Now its selling for 32 ETH, a bit above its premium price, but if the collection grows, as will the bonding curve price, or gas prices, it will become more expensive to mine this pattern.
Ultra Rare
2 greens = ~1/8680
7:2 greens = ~1/7,111,111
8:1 green = ~1/2,213,400
Connected squares
There is something aesthetic about symmetry and connected colors with these patterns. I’ll leave those calcs for another time, but every now and again, you’ll come across a pattern that you’ll need to keep. My rough numbers show a 2–10x increase in rarity for connected colors, much more so with some of the full houses combinations. Also worth looking into are full houses with 3 colors. Post your findings and corrections in the comments!
Thanks for getting this far. Shout out to Lana Ivina for helping with the calcs. If you mint a Neolastic and it’s not an ultra rare, take solace that every single pattern is unique and a lot of this is subjective. I hope this has been a fun experiment for NFTs, good luck collecting, and I look forward to seeing what new patterns emerge!
About The Project:
A few words about the project from the artist.
In November 2016, I proposed the idea of creating an autonomous artist that sells its own generative art using an early version of what would become bonding curves. A year later, I had a more concrete proposal in summoning an autonomous artist that tasked a crowd to curate a generator. In an attempt to compress these ideas into a simple MVP, I formulated a new version that directly tied newly minted pieces directly onto a bonding curve. Since then, NFTs, Generative Art, and Bonding Curves have increased in popularity and it’s time push ahead in these ideas. Thus, Neolastics seek to create a simple art project whereupon generative art is backed by a bonding curve economy. If successful, this could continue the aim of building a fully autonomous artist: eventually leading to one that even has its own ‘mind’.I’m a huge fan of Mondrian’s Neo-Plasticism art and it served as inspiration for the kind of generative art I wish to see. Hat tip to Clovers.Network for pushing the boundaries of a generative art + bonding curve economy.
About The Artist:
I’m a creator at heart. I have created games, writing, music, code, companies, and new economics. Solving the problems of the creator has always been important to me. In the past I co-founded Ujo Music, working with Grammy-winning artists such as Imogen Heap and RAC to launch the first music royalty projects using smart contracts. I’ve helped kickstart wholly new markets and economies. I helped to create the Ethereum ERC20 token standard and token bonding curves, technologies that’s currently facilitating economies worth several billion dollars of value. I enjoy creating new forms of art and experimenting with ways to empower the creative industry.
See my other art projects here: https://blog.simondlr.com/art.
Swing me a follow on Twitter! @simondlr.
Website:
